Amateur mathematician Aubrey de Grey has stunned the maths world by making the first significant progress in decades towards solving a longstanding riddle - one that's perplexed mathematical thinkers for over 60 years.
The riddle, called the Hadwiger-Nelson problem, is basically all about untouchable colours, and how many of them – or, rather, how few – can be represented on a graph with potentially infinite connections.
Picture a graph made up of a number of different scattered points on a plane, all connected by lines drawn between them. If each of these points (or vertices) were coloured, how many different colours would you need so that no two connected dots shared the same hue?
In a nutshell, that's as simple as the Hadwiger-Nelson problem is, but solving the puzzle is no easy task – especially when the question theoretically contemplates an infinite number of linked vertices.
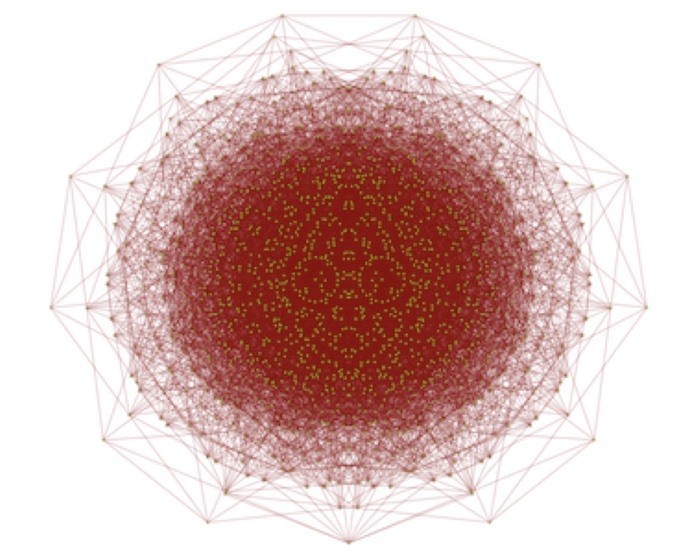De Grey's 1,581-point graph (De Grey)
First comprehensively formulated by Princeton mathematician Edward Nelson in 1950, the problem has never been definitely solved, but not for lack of trying.
Soon after the question was first posed, mathematicians figured out that an infinite Hadwiger-Nelson plane would require no fewer than four colours, but wouldn't need more than seven.
Then, for decades, minimal progress was made on narrowing this range down any further – until this month, when de Grey uploaded a new proof to the pre-print research site arXiv.org.
De Grey, who only tinkers with mathematics for fun in his spare time, isn't just notable for the new solution.
He's better known for being a provocative longevity researcher, who thinks human ageing processes can actually be reversed – and helps lead a research foundation dedicated to investigating how regenerative medicine can cure "age-related disease".
Ultimately, the group's mission is to see humans live for hundreds of years longer than our bodies currently allow – potentially to an age of 1,000, believe it or not – which is pretty heady stuff, so it's no wonder the gerontologist likes to tinker with math puzzles in his downtime.
Fortunately, as de Grey explained to Quanta Magazine, last Christmas gave him an opportunity to do just that, and by fiddling around with the Hadwiger-Nelson problem, he figured out an assumption mathematicians had made for decades was in fact quite wrong.
In his pre-print solution, de Grey demonstrates that a graph with 1,581 vertices requires at least five different colours – not four as had been previously thought to be the lower range answer to the problem.
De Grey made the discovery by playing around with a shape called the Moser spindle, composed of seven vertices and eleven edges.
By aggregating huge numbers of these constructs together with other shapes, de Grey realised a composite of 20,425 points required more than four colours: the first time the Hadwiger-Nelson range had been narrowed in more than 60 years.
Eventually, de Grey minimised his five-colour graph to 1,581 vertices, and along with sharing his new work, invited other mathematicians to see if they could improve upon this further, by finding graphs with even fewer points that require at least five colours.
A number of mathematicians have taken part in the challenge, and at present, the new record seems to be 826 vertices – but now that there's a fresh revival of interest in Hadwiger-Nelson and colours that can't touch, there's no telling how far the research could go from here.
As for de Grey, the man who thinks we'll one day live to 1,000 is pretty humble about his contribution. He told Quanta, "I got extraordinarily lucky".
The pre-print findings are available at arXiv.org.