Hi there, welcome to another dimension, where up is down, right is wrong, and squares are circles. Seriously, bring us all your squares and we'll turn them into circles for you. Just place them in front of this mirror interdimensional portal here, and voilà! You're welcome.
Meet one of the finalists of the aptly named Best Illusion of the Year Contest 2016 - the 'ambiguous cylinder illusion', performed by engineer Kokichi Sugihara from Meiji University in Japan.
So what's actually going on here? Like any good optical illusion, it's a play on perspective - our eyes see something that our brains have a difficult time interpreting and correcting.
As Sugihara explains at the Illusion of the Year website:
"The direct views of the objects and their mirror images generate quite different interpretations of the 3D shapes. … We cannot correct our interpretations, although we logically know that they come from the same objects. Even if the object is rotated in front of a viewer, it is difficult to understand the true shape of the object, and thus the illusion does not disappear."
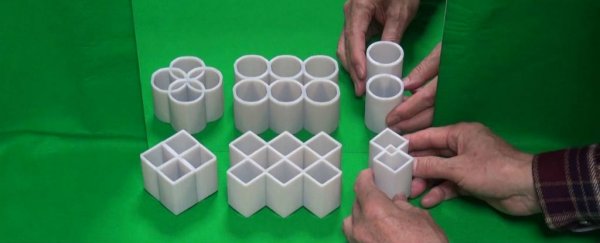
What's messing with our poor old brains here is the very tricky shape of the square, because it's not really a true square - it's more of a combination of a square and a circle, as you can see in the explainer video below by the Make Anything // 3D Printing YouTube channel.
From certain angles, those squares look pretty even, but if you pick one up and turn it around, you'll see that two of the sides curve upwards, and two of the sides curve down.
"This sort of 'corrects' the hybrid squircle into either a square or a circle, by compensating in opposite directions," says the explainer video. "So it's basically blending back into a square or blending back into a circle, when the true shape is right in between."
You can actually see evidence of this when Sugihara spins his shapes around in front of the mirror - as they turn, they look oddly warped, before looking like squares again. So, so cool.
Check out the rest of this year's finalists here, and watch the how-to video so you can see exactly how it's done:

H/T: SPLOID