When it comes to the fabled Riemann zeta function, the world's best mathematicians have suffered 160 years of dead-end after dead-end. 

But now a trio of mathematicians have discovered a new approach to solving what's been called "the greatest unsolved problem in mathematics", and it just got someone closer to a US$1 million cash prize.

First proposed in a 1859 paper by German mathematician Bernhard Riemann, the Riemann zeta function would provide us with a foolproof way to wrangle the famously unwieldy world of prime numbers - if we can prove it.

The reason prime numbers are so difficult to identify and predict is that they appear to be completely random - although there are hints that there might be some kind of order that we're yet to define.

They also contain no factors - numbers we can multiply together to get another number - other than 1 and themselves, which makes them almost frustratingly simple entities.

But what the Riemann zeta function hypothetically allows you do to is use one formula to calculate how many primes there are below any given threshold. 

It's so incredibly powerful, that over several decades, countless algorithms - especially in security and cryptography - have been formulated assuming that it's true.

If it can be proven, it will open up an entire world of new mathematics, akin to how algebraic number theory revolutionised the field before it.

But if someone figures out how to disprove Riemann's hypothesis, "the failure … would create havoc in the distribution of prime numbers", Italian number theorist Enrico Bombieri once wrote.

The reason the problem has been included as one of seven Millennium Prize Problems in mathematics that each carry a $1 million payout is because it's not only crucial that we figure out its veracity, doing so is mind-bogglingly difficult. 

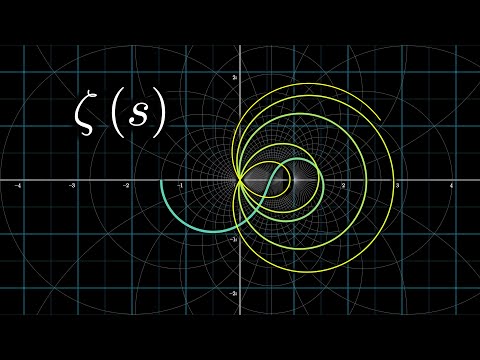
It's based on what's referred to as the Zeta Function zeroes - algorithms that begin with any two coordinates and uses those to perform a set calculation to figure out a value. 

"If you imagine the two initial coordinates to be values for latitude and longitude, for example, then the Zeta Function returns the altitude for every point, forming a kind of mathematical landscape full of hills and valleys," Matt Parker explains for The Guardian. 

"Riemann was exploring this landscape when he noticed that all of the locations that have zero altitude (points at 'sea level' in our example) lie along a straight line with a 'longitude' of 0.5 - which was completely unexpected."

Riemann used these zeroes to come up with a formula to define prime number distribution, but he was unable to prove that they all fell on the same straight line.

And not for lack of trying - you could individually prove that the first 100 billion or 10 trillion zeros all fall on that line, but what about the zeros that come after? How do you prove that infinite zeroes will still follow this trend?

Now a new paper by three mathematicians in the US, Canada, and the UK proposes that we use quantum mechanics to solve the problem, latching onto a decades-old idea that there could exist a quantum system whose energy states correspond to the hypothetical zeros of the zeta function.

They've defined a component called a Hamiltonian operator (denoted as H) as being the key to the existence of this quantum system, and the mathematics community is now buzzing over their concluding statement: 

"If the analysis presented here can be made rigorous to show that Η is manifestly self-adjoint, then this implies that the Riemann hypothesis holds true." 

To put that more simply, says Kevin Knudson at Forbes, "Should such a system exist, the Riemann Hypothesis would follow immediately. 

The hypothesis is strong enough to have gotten everyone's attention, but the jury's still out on if this is the key to unlocking what's been defined as the most important open question in pure mathematics.

Because if anything requires some serious mulling time, it's this. 

"I would need more time to give a relevant opinion about the significance of their findings as a strategy towards the Riemann hypothesis," Paul Bourgade, a mathematician at New York University, told Natalie Wolchover at Quanta Magazine.

The research has been published in Physical Review Letters, and you can find out more about Riemann zeta function below: